
NACA 4 DIGIT AIRFOIL GENERATOR MATLAB SERIES
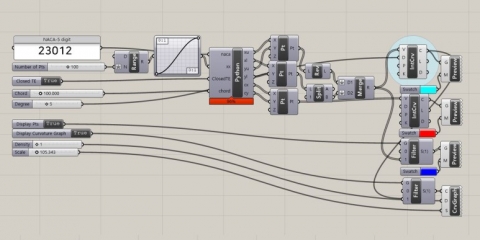
The camber line is a polynomialįunction based on the desired ideal lift coefficient.įor 6 Series Sections the designation numbers Thickness distribution is thus based on a prescribed velocity distributionįor the specific symmetric section required. Laminar flow and low drag over a reasonable range of angles of attack. These aerofoil sections are designed to produce The program below creates coordinate data that can then be stored as an ASCII formatted data file for use with other applications. X coordinate an upper (x u,y u) and lower surface (x l,y l) data point is created by applying the above equations and construction method.Ī leading edge radius r is applied to smooth the front data points. Normally generated using a cosine distribution of chord x coordinates. Surface points which are the sum of camber and thickness effects. The construction of the section is then done numerically by identifying The value of maximum camber height and its position will now be determined by the section construction process.(ie 23012 = maximum camber height =0.02c located at 0.15c). Values p, k and m are found from the following table based on the first three digits of the designation number. (ie 2412 = maximum camber height =0.02c located at 0.4c).įor the 5-digit family, the mean line is given as, m being the value of maximum camber height (1/100ths chord) and p being the position of maximum camber height (1/10ths chord). Values p and m are given from the first two digits of the designation number. Where x is a position along the chord line, given as a fraction of chord and t is the value of maximum thickness as given by the last two digits of the aerofoil designation number.(ie 0012 = symmetric section with t(max)=0.12c)įor the 4-digit family, the mean line is given as, The aerofoils are created by summing a thickness distribution with a given mean line equation.įor both families of aerofoil section the thickness distribution is as follows, While these sections are slightly out of date in terms of current aircraft usage, they still represent useful sections and are easy to The NACA 4 and 5 Digit aerofoils represent two families of aerofoil section that can be generated by the use of a set of simple polynomial equations. Systems below represent a first attempt at a parametric representation of Will have methods for determining the surface x,y coordinates, the NACA Mapping from the desired aerodynamic properties to a geometry that will then Related to the aerodynamic behaviour of the section and are constructed by More modern designation numbers, such as the 6 and 6A series

The designation numbers determine the mean line and thickness distribution of One of the earliest and simplest namingĬonventions is that for the NACA 4 and/or 5 Digit aerofoil families. Some are classified by their geometric properties while others by Aerofoil sections come in a variety of shapes and


 0 kommentar(er)
0 kommentar(er)
